Systematic sampling
In systematic sampling, individuals are chosen at regular intervals using a sampling frame to help you do this.You will recall from earlier in Section 15.2 that the sampling frame is a list of your entire study population. Figure 15.3 shows an example in which every tenth individual has been selected from the sampling frame i.e. the sampling interval is 10.
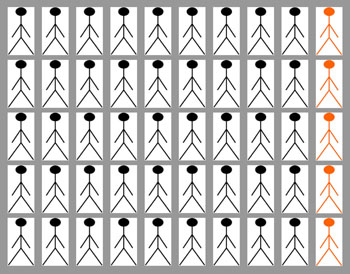
Ideally you should randomly select a number where you start selecting individuals from the list (in the example in Figure 15.3, the starting number is 1). Then, you will select your study subjects starting from that point, choosing whatever sampling interval seems appropriate. For example, you could decide to question people in every 10th house (sampling interval of 10), or selecting every 20th person (sampling interval of 20) from a list.
Systematic sampling is usually less time-consuming and easier to perform than simple random sampling. However, there is a risk of bias, as the sampling interval may accidentally coincide with a variation in the study population that you did not expect. For example, if you wanted to select a random sample of days on which to count Health Post attendance, systematic sampling with a sampling interval of seven days would be inappropriate.
Can you explain why?
All selected study days would fall on the same day of the week. If every study day was a Thursday, and Thursday is market day, then your study days would not be typical days and your sample would not be representative.
Case Study 15.2 presents an example of how to select a systematic sample. Read it carefully and then answer the question that follows it.
Case Study 15.2 A systematic sample of students
A systematic sample is to be selected from 1,200 students from the same school. The required sample size is 100. The study population is 1,200 and the sample size is 100, so a systematic sampling interval is found by dividing the study population by the sample size:
1,200 ÷ 100 = 12
The sampling interval is therefore 12.
The number of the first student to be included in the sample should be chosen randomly, for example by blindly picking one out of twelve pieces of paper, numbered 1 to 12. If number 6 is picked, then every twelfth student will be included in the sample, starting with student number 6, until 100 students have been selected.
What would the first four numbers selected be in the example in Case Study 15.2?
The first four numbers selected would be 6, 18, 30 and 42, because the first number is 6 and the next one is found by adding 12 to the previous number and so on.